ED7-5
Possibility of using the pinning phenomenon of superconductors as a reservoir computing
Dec. 1 12:30-12:45
*Ken Arita1, Tenma Ueda1, Edmund S Otabe1, Yuki Usami1, Hirofumi Tanaka1, Tetsuya Matsuno2
Kyusyu Institute of Technology (Japan)1
National Institute of Technology, Ariake College (Japan)2
Reservoir computing, which takes advantage of physical phenomena with nonlinearities, is attracting a lot of attention. In fact, such physical reservoir computing has attracted increasing attention in diverse fields of research [1]. Therefore, we turned our attention to superconductivity, a physical phenomenon with nonlinearities between the electric field and the current density. Compared to other physical phenomena used in reservoirs, we believe that superconductivity can easily adjust the nonlinearity by pin placement to produce dynamics suitable for reservoirs.
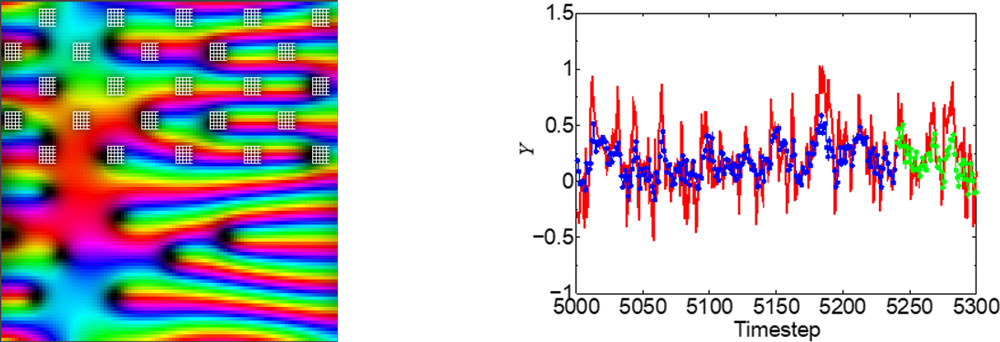
First, the time-dependent Ginzburg-Landau equation was solved numerically with Affine Integrator (AFI) [2] to visualize the motion of magnetic flux lines. The results are shown in Fig. 1. Then, the nonlinearity between the electric field generated by the motion of the magnetic flux lines and the input current density was used to perform a reservoir computing task. Three tasks were performed: a waveform generation task, a Nonlinear Autoregressive Moving Average (NARMA)2 task, and a nonlinear-memory task, and it was observed that the waveforms of the predictions followed the target waveforms. These results clearly show that superconductivity has enough important characteristics to be used as a reservoir. The results of the NARMA2 task are shown in Fig. 2. The first possible cause for the success of the tasks is the flux pinning interaction. The relationship between the E-J property of superconductivity and the pinning of magnetic flux is a well-known part of the superconductivity. This sudden generation of an electric field creates a nonlinearity. Another possible cause is the reversible motion of the magnetic flux lines. This one is mainly related to memorability. It is thought that memorability results from the inclusion of stored energy in the energy density during reversible motion inside the pin.
[1]Yuki Usami, Bram van de Ven, Dilu G Mathew, et al. Advanced Materials, 33(48):2102688, 2021.
[2] T. Matsuno, E.S. Otabe, Y. Mawatari, J.Phys. Soc. Japan 89 (2020) 054006.
Fig. 1: Motion of magnetic flux lines. The magnitude and phase of the order parameter are represented by luminance and hue.
Fig. 2: Result of the NARMA2 task. Red is the target, blue is the prediction during learning, and green is the prediction after learning.
Keywords: Reservoir computing, Nonlinearity, Pinning